Jump to content

Kondo effect

From Wikipedia, the free encyclopedia
Kondo effect: How gold with a small amount of what were probably iron impurities behaves at low temperatures
Jun Kondo

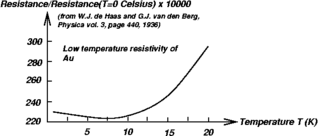
In physics, the Kondo effect describes the scattering of conduction electrons in a metal due to magnetic impurities, resulting in a characteristic change i.e. a minimum in electrical resistivity with temperature.[1] The cause of the effect was first explained by Jun Kondo, who applied third-order perturbation theory to the problem to account for scattering of s-orbital conduction electrons off d-orbital electrons localized at impurities (Kondo model). Kondo's calculation predicted that the scattering rate and the resulting part of the resistivity should increase logarithmically as the temperature approaches 0 K.[2] Extended to a lattice of magnetic impurities, the Kondo effect likely explains the formation of heavy fermions and Kondo insulators in intermetallic compounds, especially those involving rare earth elements such as cerium, praseodymium, and ytterbium, and actinide elements such as uranium. The Kondo effect has also been observed in quantum dot systems.

Theory

[edit]

The dependence of the resistivity on temperature , including the Kondo effect, is written as

where is the residual resistivity, the term shows the contribution from the Fermi liquid properties, and the term is from the lattice vibrations: , , and are constants independent of temperature. Jun Kondo derived the third term with logarithmic dependence on temperature and the experimentally observed concentration dependence.

History

[edit]

In 1930, Walther Meissner and B. Voigt[3][4] observed that the resistivity of nominally pure gold reaches a minimum at 10 K, and similarly for nominally pure Cu at 2 K. Similar results were discovered in other metals.[5] Kondo described the three puzzling aspects that frustrated previous researchers who tried to explain the effect:[6][7]

  • The resistivity of a truly pure metal is expected to decrease monotonically, because with lower temperature, the probability of electron-phonon scattering decreases.
  • The resistivity should rapidly plateau when the temperature drops below the Debye temperature of the phonons, below which the phonons.[clarification needed] However, in the AuFe alloy, the resistivity continues to rise sharply below 0.01 K, yet there seemed to be no energy gap in AuFe alloy that small.
  • The phenomenon is universal, so any explanation should apply in general.

Experiments in the 1960s by Myriam Sarachik at Bell Laboratories showed that phenomenon was caused by magnetic impurity in nominally pure metals.[8] When Kondo sent a preview of his paper to Sarachik, Sarachik confirmed the data fit the theory.[9]

Kondo's solution was derived using perturbation theory resulting in a divergence as the temperature approaches 0 K, but later methods used non-perturbative techniques to refine his result. These improvements produced a finite resistivity but retained the feature of a resistance minimum at a non-zero temperature. One defines the Kondo temperature as the energy scale limiting the validity of the Kondo results. The Anderson impurity model and accompanying Wilsonian renormalization theory were an important contribution to understanding the underlying physics of the problem.[10] Based on the Schrieffer–Wolff transformation, it was shown that the Kondo model lies in the strong coupling regime of the Anderson impurity model. The Schrieffer–Wolff transformation[11] projects out the high energy charge excitations in the Anderson impurity model, obtaining the Kondo model as an effective Hamiltonian.

Schematic of the weakly coupled high temperature situation in which the magnetic moments of conduction electrons in the metal host pass by the impurity magnetic moment at speeds of vF, the Fermi velocity, experiencing only a mild antiferromagnetic correlation in the vicinity of the impurity. In contrast, as the temperature tends to zero the impurity magnetic moment and one conduction electron moment bind very strongly to form an overall non-magnetic state.

The Kondo effect can be considered as an example of asymptotic freedom, i.e. a situation where the coupling becomes non-perturbatively strong at low temperatures and low energies. In the Kondo problem, the coupling refers to the interaction between the localized magnetic impurities and the itinerant electrons.

Examples

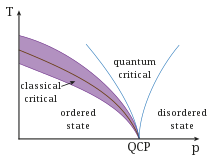
[edit]

Extended to a lattice of magnetic ions, the Kondo effect likely explains the formation of heavy fermions and Kondo insulators in intermetallic compounds, especially those involving rare earth elements such as cerium, praseodymium, and ytterbium, and actinide elements such as uranium. In heavy fermion materials, the non-perturbative growth of the interaction leads to quasi-electrons with masses up to thousands of times the free electron mass, i.e., the electrons are dramatically slowed by the interactions. In a number of instances they are superconductors. It is believed that a manifestation of the Kondo effect is necessary for understanding the unusual metallic delta-phase of plutonium.[citation needed]

The Kondo effect has been observed in quantum dot systems.[12][13] In such systems, a quantum dot with at least one unpaired electron behaves as a magnetic impurity, and when the dot is coupled to a metallic conduction band, the conduction electrons can scatter off the dot. This is completely analogous to the more traditional case of a magnetic impurity in a metal.

Band-structure hybridization and flat band topology in Kondo insulators have been imaged in angle-resolved photoemission spectroscopy experiments.[14][15][16]

In 2012, Beri and Cooper proposed a topological Kondo effect could be found with Majorana fermions,[17] while it has been shown that quantum simulations with ultracold atoms may also demonstrate the effect.[18]

In 2017, teams from the Vienna University of Technology and Rice University conducted experiments into the development of new materials made from the metals cerium, bismuth and palladium in specific combinations and theoretical work experimenting with models of such structures, respectively. The results of the experiments were published in December 2017[19] and, together with the theoretical work,[20] lead to the discovery of a new state,[21] a correlation-driven Weyl semimetal. The team dubbed this new quantum material Weyl-Kondo semimetal.

References

[edit]
  1. ^ Hewson, Alex C; Jun Kondo (2009). "Kondo effect". Scholarpedia. 4 (3): 7529. Bibcode:2009SchpJ...4.7529H. doi:10.4249/scholarpedia.7529.
  2. ^ Kondo, Jun (1964). "Resistance Minimum in Dilute Magnetic Alloys". Progress of Theoretical Physics. 32 (1): 37–49. Bibcode:1964PThPh..32...37K. doi:10.1143/PTP.32.37.
  3. ^ Meissner, W.; Voigt, B. (January 1930). "Messungen mit Hilfe von flüssigem Helium XI Widerstand der reinen Metalle in tiefen Temperaturen". Annalen der Physik. 399 (7): 761–797. Bibcode:1930AnP...399..761M. doi:10.1002/andp.19303990702. ISSN 0003-3804.
  4. ^ Meissner, W.; Voigt, B. (January 1930). "Messungen mit Hilfe von flüssigem Helium XI Widerstand der reinen Metalle in tiefen Temperaturen". Annalen der Physik. 399 (8): 892–936. Bibcode:1930AnP...399..892M. doi:10.1002/andp.19303990803. ISSN 0003-3804.
  5. ^ de Haas, W. J.; de Boer, J.; van dën Berg, G. J. (1934-05-01). "The electrical resistance of gold, copper and lead at low temperatures". Physica. 1 (7): 1115–1124. Bibcode:1934Phy.....1.1115D. doi:10.1016/S0031-8914(34)80310-2. ISSN 0031-8914.
  6. ^ Kondo, J. (1970-01-01), Seitz, Frederick; Turnbull, David; Ehrenreich, Henry (eds.), Theory of Dilute Magnetic Alloys, Solid State Physics, vol. 23, Academic Press, pp. 183–281, doi:10.1016/S0081-1947(08)60616-5, ISBN 978-0-12-607723-0, retrieved 2024-06-01
  7. ^ Kondo, Jun (January 2005). "Sticking to My Bush". Journal of the Physical Society of Japan. 74 (1): 1–3. Bibcode:2005JPSJ...74....1K. doi:10.1143/JPSJ.74.1. ISSN 0031-9015.
  8. ^ Sarachik, M. P.; Corenzwit, E.; Longinotti, L. D. (1964-08-17). "Resistivity of Mo-Nb and Mo-Re Alloys Containing 1% Fe". Physical Review. 135 (4A): A1041–A1045. Bibcode:1964PhRv..135.1041S. doi:10.1103/PhysRev.135.A1041.
  9. ^ Chang, Kenneth (August 31, 2020). "Myriam Sarachik Never Gave Up on Physics". The New York Times. ISSN 0362-4331. Archived from the original on August 31, 2020. Retrieved October 13, 2021.
  10. ^ Anderson, P. (1961). "Localized Magnetic States in Metals" (PDF). Physical Review. 124 (1): 41–53. Bibcode:1961PhRv..124...41A. doi:10.1103/PhysRev.124.41.[permanent dead link]
  11. ^ Schrieffer, J.R.; Wolff, P.A. (September 1966). "Relation between the Anderson and Kondo Hamiltonians". Physical Review. 149 (2): 491–492. Bibcode:1966PhRv..149..491S. doi:10.1103/PhysRev.149.491. S2CID 55838235.
  12. ^ Cronenwett, Sara M. (1998). "A Tunable Kondo Effect in Quantum Dots". Science. 281 (5376): 540–544. arXiv:cond-mat/9804211. Bibcode:1998Sci...281..540C. doi:10.1126/science.281.5376.540. PMID 9677192. S2CID 5139144.
  13. ^ "Revival of the Kondo" (PDF). Archived from the original (PDF) on 2017-05-17. Retrieved 2016-08-19. {{cite journal}}: Cite journal requires |journal= (help)
  14. ^ Neupane, Madhab; Alidoust, Nasser; Belopolski, Ilya; Bian, Guang; Xu, Su-Yang; Kim, Dae-Jeong; Shibayev, Pavel P.; Sanchez, Daniel S.; Zheng, Hao; Chang, Tay-Rong; Jeng, Horng-Tay; et al. (2015-09-18). "Fermi surface topology and hot spot distribution in the Kondo lattice system CeB6". Physical Review B. 92 (10): 104420. arXiv:1411.0302. Bibcode:2015PhRvB..92j4420N. doi:10.1103/PhysRevB.92.104420.
  15. ^ Neupane, M.; Alidoust, N.; Xu, S.-Y.; Kondo, T.; Ishida, Y.; Kim, D. J.; Liu, Chang; Belopolski, I.; Jo, Y. J.; Chang, T.-R.; Jeng, H.-T. (2013). "Surface electronic structure of the topological Kondo-insulator candidate correlated electron system SmB6". Nature Communications. 4 (1): 2991. arXiv:1312.1979. Bibcode:2013NatCo...4.2991N. doi:10.1038/ncomms3991. ISSN 2041-1723. PMID 24346502.
  16. ^ Hasan, M. Zahid; Xu, Su-Yang; Neupane, Madhab (2015), "Topological Insulators, Topological Dirac semimetals, Topological Crystalline Insulators, and Topological Kondo Insulators", Topological Insulators, John Wiley & Sons, Ltd., pp. 55–100, doi:10.1002/9783527681594.ch4, ISBN 978-3-527-68159-4, retrieved 2020-04-26
  17. ^ Béri, B.; Cooper, N. R. (2012). "Topological Kondo Effect with Majorana Fermions". Physical Review Letters. 109 (15): 156803. arXiv:1206.2224. Bibcode:2012PhRvL.109o6803B. doi:10.1103/PhysRevLett.109.156803. PMID 23102351. S2CID 45712589.
  18. ^ Buccheri, F.; Bruce, G. D.; Trombettoni, A.; Cassettari, D.; Babujian, H.; Korepin, V. E.; Sodano, P. (2016-01-01). "Holographic optical traps for atom-based topological Kondo devices". New Journal of Physics. 18 (7): 075012. arXiv:1511.06574. Bibcode:2016NJPh...18g5012B. doi:10.1088/1367-2630/18/7/075012. ISSN 1367-2630. S2CID 118610269.
  19. ^ Dzsaber, S.; Prochaska, L.; Sidorenko, A.; Eguchi, G.; Svagera, R.; Waas, M.; Prokofiev, A.; Si, Q.; Paschen, S. (2017-06-16). "Kondo Insulator to Semimetal Transformation Tuned by Spin-Orbit Coupling". Physical Review Letters. 118 (24): 246601. arXiv:1612.03972. Bibcode:2017PhRvL.118x6601D. doi:10.1103/PhysRevLett.118.246601. ISSN 0031-9007. PMID 28665644.
  20. ^ Lai, H.H.; Grefe, S.E.; Paschen, S.; Si, Q. (2012). "Weyl–Kondo semimetal in heavy-fermion systems". Proceedings of the National Academy of Sciences of the United States of America. 115 (1): 93–97. arXiv:1206.2224. Bibcode:2018PNAS..115...93L. doi:10.1073/pnas.1715851115. PMC 5776817. PMID 29255021.
  21. ^ Gabbatiss, J. (2017) "Scientists discover entirely new material that cannot be explained by classical physics", The Independent

Further reading

[edit]
  • Kondo Effect - 40 Years after the Discovery - special issue of the Journal of the Physical Society of Japan
  • Kondō, Jun (2012). The physics of dilute magnetic alloys. Cambridge ; New York: Cambridge University Press. ISBN 978-1-107-02418-2.. Monograph by Kondo himeslf.
  • Hewson, Alexander C. (2003). The Kondo problem to heavy fermions. Cambridge studies in magnetism (1. paperback ed. (with corr.), transferred to digital printing 2003 ed.). Cambridge: Cambridge Univ. Press. ISBN 978-0-521-59947-4.
  • Cox, D. L.; Zawadowski, A. (1999). Exotic Kondo effects in metals: magnetic ions in a crystalline electric field and tunnelling centres. London: Taylor & Francis. ISBN 978-0-7484-0889-4. Monograph on newer versions of the Kondo effect in non-magnetic contexts especially
Retrieved from "https://en.wikipedia.org/w/index.php?title=Kondo_effect&oldid=1233664418"